A team of researchers from the University of Ottawa’s Nexus for Quantum Technologies Institute (NexQT), led by Dr. Francesco Di Colandrea, under the supervision of Professor Ebrahim Karimi, associate professor of physics, has developed an innovative technique for evaluating the performance of quantum circuits. This significant advancement, recently published in the prestigious journal npj Quantum Information, represents a substantial leap forward in the field of quantum computing.
In the rapidly evolving landscape of quantum technologies, ensuring the functionality and reliability of quantum devices is critical. The ability to characterize these devices with high accuracy and speed is essential for their efficient integration into quantum circuits and computers, impacting both fundamental studies and practical applications.
Characterization helps determine if a device operates as expected, which is necessary when devices exhibit anomalies or errors. Identifying and addressing these issues is crucial for advancing the development of future quantum technologies.
Traditionally, scientists have relied on Quantum Process Tomography (QPT), a method that requires a large number of “projective measurements” to reconstruct a device’s operations fully. However, the number of required measurements in QPT scales quadratically with the dimensionality of the operations, posing significant experimental and computational challenges, especially for high-dimensional quantum information processors.
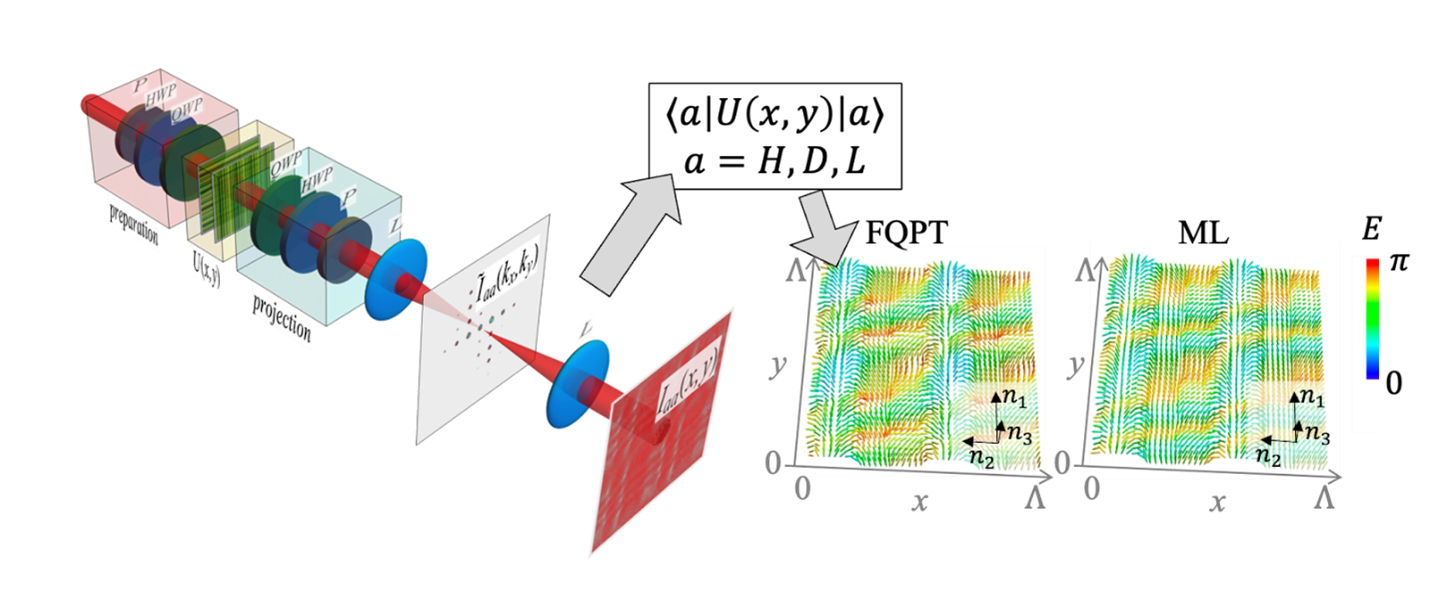
The University of Ottawa research team has pioneered an optimized technique named Fourier Quantum Process Tomography (FQPT). This method allows for the complete characterization of quantum operations with a minimal number of measurements. Instead of performing a large number of projective measurements, FQPT utilises a well-known map, the Fourier transform, to perform a portion of the measurements in two different mathematical spaces. The physical relation between these spaces enhances the information extracted from single measurements, significantly reducing the number of measurements needed. For instance, for processes with dimensions 2d (where d can be arbitrarily high), only seven measurements are required.
To validate their technique, the researchers conducted a photonic experiment using optical polarisation to encode a qubit. The quantum process was realized as a complex space-dependent polarisation transformation, leveraging state-of-the-art liquid-crystal technology. This experiment demonstrated the flexibility and robustness of the method.
“The experimental validation is a fundamental step to probe the technique’s resilience to noise, ensuring robust and high-fidelity reconstructions in realistic experimental scenarios,” said Francesco Di Colandrea, a postdoctoral fellow at the University of Ottawa.
This novel technique represents a remarkable advancement in quantum computing. The research team is already actively working on extending FQPT to arbitrary quantum operations, including non-Hermitian and higher-dimensional implementations, and in implementing AI techniques to increase accuracy and reduce measurement. This new technique represents a promising avenue for further advancements in quantum technology.
The study “Fourier quantum process tomography” by F Di Colandrea, N Dehghan, A D’Errico, and E Karimi was published in npj Quantum Information on May 9, 2024.
For additional information: [email protected]
